Electric_Fields

Introduction. A charge in empty space experiences no electric forces. However, a charge placed near another charge does experience electric forces. The reason for this difference is that a charge creates an electric field that permeates the surrounding space and affects other nearby charges. As long as no other charge is present, an electric field does nothing. When another charge (referred to as a test charge) is introduced into the field, the field exerts a force on it. The presence of an electric field can be detected by its effect on a charged object introduced into the region.

Electric Field Intensity. Fields, like forces, are vector quantities: they possess magnitude and direction. The magnitude of an electric field is referred to as its intensity. The intensity of an electric field at a particular point is defined as the magnitude of the force that the field exerts on a test charge of one coulomb at that point. The direction of the electric field at any given point is defined as the direction of the force that the field exerts on a positive test charge at that point. The intensity and direction of an electric field are different at different positions in the vicinity of the charge, and therefore, the magnitude and direction of the electric force also vary from point to point.

The electric field intensity E at a particular point is given by the relationship

E = Fe/q

where Fe is the force (N) exerted on a test charge q (C). The unit for electric field intensity is N/C.

If the electric field intensity at a particular point is known, the force exerted on any amount of charge at that point is

Fe = qE

Electric Field Diagrams. A representation of the field around a single point charge appears as arrows radiating either inward towards the center or outward from the center. These lines are referred to as field lines. By convention, field lines always point in the direction of the force that would be exerted by that field on a positive test charge. The concentration of field lines at any point in a field diagram indicates the intensity of the field. The intensity of an electric field decreases inversely with the square of the distance from a point charge. Thus, the number of field lines per unit area (perpendicular to the field's direction) also decreases inversely with the square of the distance from the point charge. At a point twice as far from the charge, the concentration of field lines per unit area is one-fourth as great, as is the intensity of the field. Although the field lines in the vicinity of a negative point charge are directed radially inwards, they possess the same characteristics as the field lines around a positive point charge. Field lines are always oriented at right angles to the surface(s) of the charged object(s).

At any point within a charged hollow spherical body, the field intensity is zero. The net force on a test charge, found by adding the forces exerted on all the charges on the hollow sphere, is zero. Therefore, there is no electric field inside the hollow sphere. Outside the hollow sphere, the field in the same as though all the charges were concentrated at the center of the hollow sphere.

The field created by two equal positive point charges is the same in appearance as that created by two equal negative point charges except in the orientation of the arrows: outward from the positive point charges and inward towards the negative point charges. The field lines in the space directly between the pair of identical point charges are deflected laterally and never mutually cross.

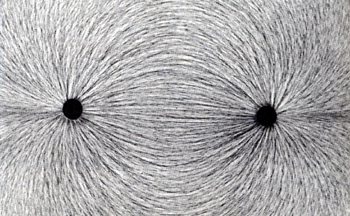
The field created by two equal but opposite point charges appears as a single straight and a set of curved lines extending radially outward from the positive point charge and ending radially inward at the negative point charge.

The electric field lines between two parallel but oppositely charged plates generally appear as parallel lines extending perpendicularly from the positive plate and ending at the negative plate. In the case where the distance between the plates is small compared to their size and the charge of the plates is uniformly distributed, the field lines are parallel to each other and perpendicular to the plates (except near the edges where they bulge slightly outward). If a positive test charge is released between the plates, it will be pushed in a straight line from the positive plate to the negative plate. Since the field between the plates is uniformly intense, the magnitude of the force exerted on a test charge is the same everywhere in the region between the plates (except near the edges). Outside the plates, the net force is practically zero, so no fieldlines appear there.

The following features are common to all electric field diagrams:

a. Field lines always begin on the surfaces of positively charged objects and terminate on the surfaces of negatively charged objects.

b. Field lines never intersect one another.

c. Where field lines meet a charged object they are oriented perpendicularly to the surface of the charged object.

d. Field lines do not extend below the surface of a charged object.

Electric Potential Energy. The electric force, like the gravitational force, can push or pull an object through a distance and thereby do work. Just as a mass has gravitational potential energy because of its position in a gravitational field, a charged object has electrical potential energy due to its position in an electric field.

If an electric field does work on any charged object, such as when the field created by one positive charge repels another positive charge, electric potential energy decreases. Similarly, electric potential energy decreases when the field created by a positive charge attracts a negative charge, pulling the charges closer together. This is analogous to a falling object on Earth. When the gravitational field does work, gravitational potential energy does work, and gravitational potential energy decreases. In accordance with the Law of Conservation of Energy, the lost potential energy is converted into an equal amount of energy of another type, such as kinetic energy or heat.

If work is done against an electric field, such as when a positive test charge is moved closer to another positive charge, electric potential energy increases. Similarly, electric potential energy increases when a negative charge is moved away from a positive charge. This is analogous to the lifting of an object on Earth. When work is done against the gravitational field, gravitational potential energy increases. Once again, the gain in potential energy must correspond to a loss of energy of some other type, in order that energy be conserved. The work done by (or against) an electric field in moving a charge from one point to another is independent of the path taken. In other words, the electric force is a conservative force, as is gravity.

Electric Potential. The electric potential at any given point in an electric field is defined as the total amount of work required to bring one coulomb of positive charge from infinity to that point. At a point in a field created by a positive charge, the force is repulsive. Thus, the work done against the field in moving the positive test charge from infinity to that point is positive. The closer the point is to the positive charge that initiates the field, the greater the electric potential.

At a point in a field created by a negative charge, the force is attractive. Thus, the work done in moving the positive test charge from infinity to the point is negative. (The field does work on the charge.) The closer the point is to the negative charge that initiated the field, the smaller the electric potential.

In summary, electric potential at any given point in an electric field is positive if work must be done against the field to move a positive test charge from infinity to that point, and negative if work is done by the the field to move a positive test charge from infinity to that point.

Potential Difference. The work required to move a test charge of one coulomb from one point to another in an electric field is equal to the difference in the electric potential between the two points. We refer to this difference as the potential difference between the two points.

From the amount of work W, in joules, required to move a charge q, in coulombs, between two points in an electric field, the potential difference V between those two points can be obtained by using the equation\

V = W/q

Thus, the potential difference is expressed in joules per coulomb (J/C). One joule per coulomb is more commonly referred to as one volt. Since the potential difference between two points is measured in volts, it is also known as the voltage.

If it takes 6 J of work to push 3 C of charge from one point to another in an electric field, the potential difference between the two points is 6 J/3 C, or 2 V. If a manufacturer labels a battery 9 V, it means that the electric field created by the two charged terminals will do 9 joules of work on every coulomb that is pushed by the battery from one terminal to the other. Since the quantity of work done is independent of the path taken between the terminals, that characteristics of the materials and appliances connected to the battery play no role in determining the voltage.

If the voltage between two points is known, the work done in moving any given amount of charge between those points can be found by the equation

W = qV

The Electron Volt. A convenient use of work and energy when working with small charges, such as protons and electron, is the electron volt (eV).One electron volt is defined as the work required to move one elementary charge between two points with a 1 volt potential difference between them. Using W = qV, the amount of work is 1.60 x 10exp-19 J. One electron volt, therefore, is equivalent to 1.60 x 10exp-19 J. When this amount of work is done on a charge, the charge gains 1 eV of energy.

Electric Field Intensity and Potential Difference. The potential difference V between two points in an electric field of uniform intensity E, such as the uniform field between two oppositely charged parallel plates, satisfies the relationship

V = Ed

where d is the distance in meters between the two points.

Solving for E we obtain

E = V/d

Thus, a uniform electric field intensity can be expressed in volts per meter (V/m). Since E was defined earlier in units of newtons per coulomb (N/C), we can conclude that 1 V/m is equivalent to 1 N/C.

________________________________

Questions.

1. Calculate the charge possessed by two objects q1 and q2 with values of -12 C and +5 C after they are connected with a conducting wire.

2. Calculate the Fe between two charges each possessing a value of -5 C and separated by a distance of 5 m.

3. Calculate the E at a distance of 2 m when a test charge of +3.20 x 10exp-15 C is placed in the field produced by a charge of +1.60 C.

4. Calculate the amount of work done in moving +5 C across a potential difference of 440 volts.

5. Calculate the number of electrons which pass a given point in a wire which is experiencing a current of 20 amperes over a 1 hour time period.

6. Assuming a uniform, steady state flow of current in a wire which only permits one electron to exit at a time, calculate the amount of time it takes between successive electrons to exit the wire if it is experiencing a current of 6 amperes.